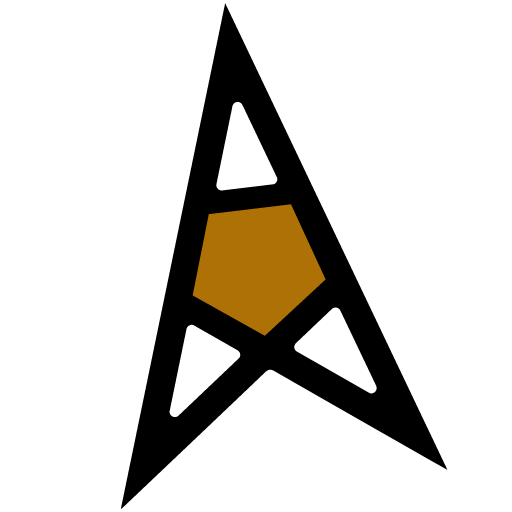
BEHOLD THE GOLDEN RATIO!
The ancient secret brought to life with 21st century technologies!
Very cool.
I’ve written about ratios and relationships before, and the thing I’m holding is my favorite ratio.
The challenge here is to explain why without getting too deep in the weeds with it.
Let’s Try
Back in ancient Greek times there were two competing theories for how the universe works.
- Everything can be reduced to its most basic building block, the atom. The atom here is the universally useful common denominator between all things in the universe (hence the use of the term universally useful).
- The whole universe is full of an odorless, colorless, weightless “aether.” There are no fundamental particles; it’s all forces transmitted through this invisible gas.
That’s it.
SIDENOTE: In all seriousness; the debate is still going thousands of years later. The atomists think they’ve won, but they don’t know they’ve backed themselves into a corner. But I digress.
The people who supported the first argument (we’ll call them ‘Atomists’ like I already did) had every reason to believe that you could find the universal building block.
Let’s build their case using some good imaginary lines. We won’t even have to draw them!
Divisibility
Imagine you have two lengths of string. One is two inches long. The other is three inches long.
There’s no way that you can squish three inches to fit into two inches, and there’s no way to stretch two into three.
What you can do, however, is cut the two inch string into two one-inch pieces. You can also cut the three inch string into three one-inch pieces.
Now you have 5 pieces of string that are all the same length.
As a result you would say that one inch is the common unit that is the same to both pieces of string.
This is (almost) universally true. No matter what units you measure by, you can (almost) always find a way to cut up both lengths that will leave you with the same size pieces.
The ancient Greeks were proving this left and right. They were using this information to find the area of planes and solids. They were calculating the center of gravity for three dimensional solids.
Life was good.
And Then Along Came Phi
Everything was great until it wasn’t.
Imagine you’ve spent a lifetime proving that you can always divide string, lines, and measurements into smaller pieces to find their common “atom” size.
And then someone shows you that doesn’t work.
It would be like finding out your tidy explanation for life, the universe, and everything has a giant black hole at the center that’s devouring everything right in front of you.
Warning: This knowledge got people killed in ancient Greece times, and there are people who have been driven mad by it much more recently because their minds simply can’t accept what this means. You have been warned.
Imagine there was a length of string that you cut into two pieces. Now, when you try to cut it down into inches you realize that there’s always going to be some nubbin left over in one of the pieces.
So the inch doesn’t work as the “atomic” denominator between them.
You decide to try centimeters. You cut both pieces into one centimeter pieces, and there’s still a little nubbin left over.
This means that the centimeter is not the atomic denominator between these two lengths of string.
Your eyes narrow.
You decide to cut both pieces into millimeter length sections.
This will do it for sure!
Nope.
There’s still a suuuuuuuper tiny left over piece. It’s less than a millimeter long, but it’s sitting right there mocking you.
That’s how you know the millimeter is not a common unit of measurement between the lengths.
This is where the nightmare truly starts.
No matter how short the length you use to cut the string, you realize there’s always going to be something left over.
What you’ve just discovered is the monster that eats the atom known as irrational numbers.
You Already Love Them
One of the most famous irrational numbers is Pi; the number that helps you compute the circumference of a cirle.
Tau is lesser known irrational number which is 2pi, and I think you’ve earned a momentary distraction from you existential dread with this wonderful talk by Michael Hartl about Tau:
And, finally, I want to tell you about Phi.
That’s what I’m holding in the photo at the top of the post. Since you’ve been reading for a long ass time already, here it is again to remind you:

Yeah, that’s what it is. But what does it mean? What are you really looking at?
The piece, at large, is an obtuse triangle with one unique angle of 108 degrees, and two shared angles of 36 degrees.
The photo makes it look very distorted. I angled it so that the light would reflect off it. Here is a straight-on shot to minimize distortion. I’ve also added dots to reference later.
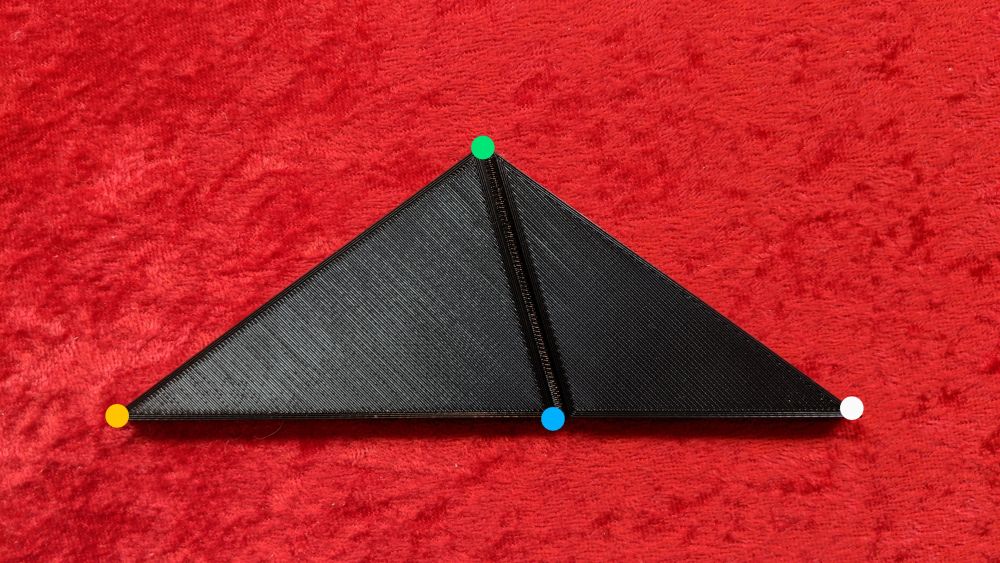
The yellow/green/white angle is 108 degrees. The green/white/blue angle is 36. The green/yellow/blue angle is 36, too.
Here’s what’s neat.
The green/blue line divides the yellow/green/white triangle into a smaller green/blue/white triangle with the same 108/36/36 angles.
Very cool.
You could continue to cut the smaller triangle into a smaller 108/36/36 triangle down to infinity.
While that stays the same, here’s what will eventually drive you mad.
The blue dot cuts the yellow/white line into two sections (yellow/blue and blue/white).
These two sections have no common denomenator.
There is no way to divide these two up without having something left over.
The segments will never find commonality.
This is provable. It’s demonstrable.
There are simply some things that will never be reduced to their essence.
There are things we can know. Things we can know by inference from others.
And some that will remain a mystery forever.
21st Century
That’s the quick outline of what the “Golden Triangle” (or better known as the “Golden Ratio”) is, and how it was discovered.
The implications are far-reaching, and much too big for this already-too-long post.
Let’s wrap this thing up by talking about how I created it.
Late at night on Twitter I asked if anyone knew how to make this idea of mine happen, and within 30 minutes I had a working file in my inbox that I could use with my 3D printer.
The next morning I ran the file through my Ender3 to make the piece I’m showing in the photos.
3D printers use a highly sophisticated Cartesian coordinate system to track its position within the context of three planes of motion.
- Forward/Back
- Left/Right
- Up/Down
Voila. The three dimensions of printing!
So using these very straightforward (even though they’re complex to execute, they’re pretty simple in practice) processes to create a physical object that embodies a fundamental truth of the universe.
Today’s post is brought to you by the word: Incommensurability